Isogeometric analysis research
For more than 15 years, thousands of papers have been published advancing the field of isogeometric analysis, and published isogeometric analysis research is growing exponentially. Key themes are emerging.
From research to commercial application
IGA has matured significantly since the first IGA paper was introduced in 2005 by T.J.R. Hughes, J.A. Cottrell, and Y. Bazilevs, becoming one of the hottest research fields in FEA and computer graphics. Many key breakthroughs and benchmarks have been established both in industry and academia.
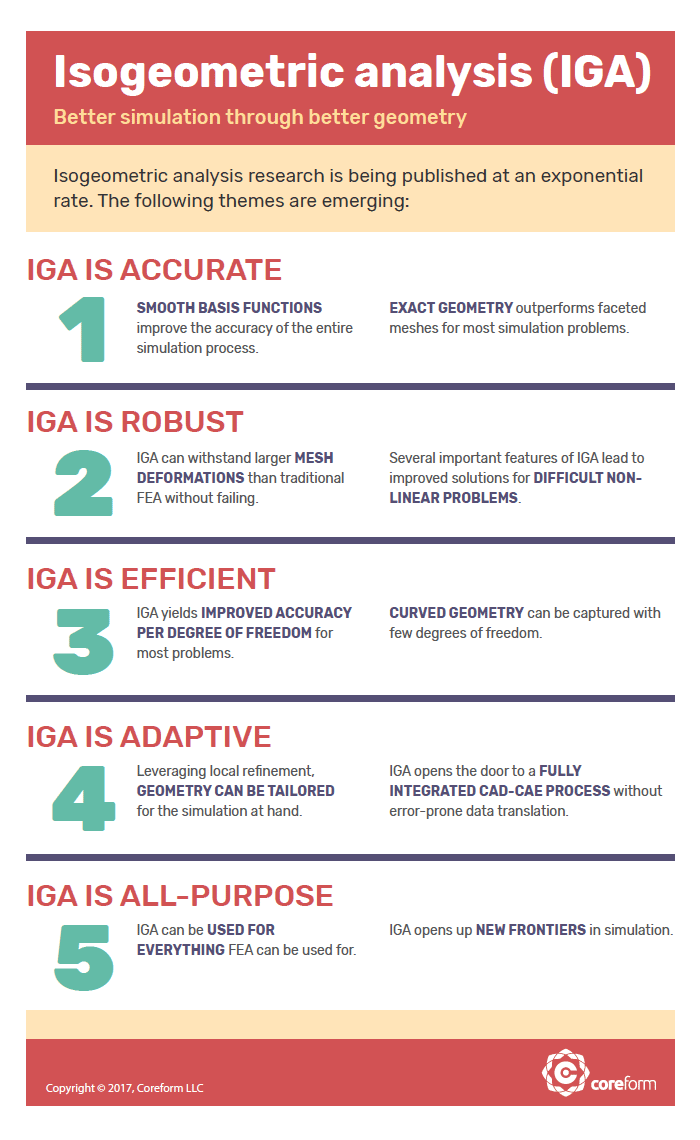
Five key descriptions of IGA are emerging from the research: accurate, robust, efficient, adaptive, and all-purpose. Below is a list of just some of the significant IGA papers grouped by these themes. We will continue to flesh out this list, please contact us if there are papers you’d like to make sure we include.
Accurate
Higher-order smooth basis functions — Smooth higher-order basis functions improve the accuracy of the entire simulation process.
- Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement.
T.J.R. Hughes, J.A. Cottrell, Y. Bazilevs - Studies of refinement and continuity in isogeometric structural analysis.
J.A. Cottrell, T.J.R. Hughes, A. Reali
Exact analysis-suitable geometry — Exact analysis-suitable CAD geometry outperforms faceted meshes for many classes of problems (i.e., contact, interface problems, etc.) and can be used in a simulation without any geometry clean-up or mesh generation steps.
- Contact treatment in isogeometric analysis with NURBS.
İ. Temizer, P. Wriggers, T.J.R. Hughes
Robust
Accommodates severe mesh deformation without remeshing — Smooth higher-order basis functions can withstand larger mesh deformations than traditional FEA without failing.
- Robustness of isogeometric structural discretizations under severe mesh distortion.
S. Lipton, J.A. Evans, Y. Bazilevs, T. Elguedj, T.J.R. Hughes
Superior non-linear behavior — Several important features of IGA lead to improved performance on hard, non-linear problems, including (i) improved spectral behavior, leading to larger time steps; (ii) basis function properties which reduce the iterations required for convergence; and (iii) exactly represented contact geometry, which eliminates spurious pressure oscillations.
- Patient-specific isogeometric structural analysis of aortic valve closure.
S. Morgantia, F. Auricchio, D.J. Benson, F.I. Gambarin, S. Hartmann, T.J.R. Hughes, A. Reali - Isogeometric shell analysis: The Reissner–Mindlin shell.
D.J. Benson, Y. Bazilevs, M.C. Hsu, T.J.R. Hughes
Efficient
Greater accuracy per degree of freedom — For many classes of problems, smooth higher-order basis functions lead to improved accuracy per degree of freedom (DOF) and highly optimized solution strategies (i.e., collocation, reduced quadrature schemes, etc.)
- Finite element and NURBS approximations of eigenvalue, boundary-value, and initial-value problems.
Thomas J.R. Hughes, John A. Evans, Alessandro Reali - Isogeometric analysis of structural vibrations.
J.A. Cottrell, A. Reali, Y. Bazilevs, T.J.R. Hughes
Lean geometry representation — Curved geometry can be captured with few degrees of freedom, leading to compact, analysis-suitable CAD representations.
- Isogeometric analysis using T-splines.
Y. Bazilevs, V.M. Calo, J.A. Cottrell, J.A. Evans, T.J.R. Hughes, S. Lipton, M.A. Scott, T.W. Sederberg
Adaptive
Geometrically exact local mesh adaptivity — Analysis-suitable CAD possesses a wide range of localized, geometrically exact, mesh adaptivity algorithms (e.g., in h (element size), p (polynomial degree), and k (smoothness)) to enable the user to tailor the geometry for the simulation at hand and reduce compute costs.
- Hierarchically refined and coarsened splines for moving interface problems, with particular application to phase-field models of prostate tumor growth.
G. Lorenzo, M.A. Scott, K. Tew, T.J.R. Hughes, H. Gomez - A phase-field description of dynamic brittle fracture.
Michael J. Borden, Clemens V. Verhoosel, Michael A. Scott, Thomas J.R. Hughes, Chad M. Landis - Local refinement of analysis-suitable T-splines.
M.A. Scott, X. Li, T.W. Sederberg, T.J.R. Hughes
Integrated design iteration — Leveraging analysis-suitable CAD opens the door to a fully integrated CAD-CAE process without the traditional time-intensive and error-prone data translation steps, like mesh generation and geometry cleanup.
- Acoustic isogeometric boundary element analysis.
R.N. Simpson, M.A. Scott, M. Taus, D.C. Thomas, H. Liane - Isogeometric structural shape optimization.
Wolfgang A. Wall, Moritz A. Frenzel, Christian Cyron - An isogeometric design-through-analysis methodology based on adaptive hierarchical refinement of NURBS, immersed boundary methods, and T-spline CAD surfaces.
Dominik Schillinger, Luca Dedè, Michael A. Scott, John A. Evans, Michael J. Borden, Ernst Rank, Thomas J.R. Hughes - A rapid and efficient isogeometric design space exploration framework with application to structural mechanics.
J. Benzaken, A.J. Herrema, M.-C. Hsu, J.A. Evans
All-purpose
IGA can be used for everything FEA can be used for — IGA is a generalization of FEA, so problems that can be solved with FEA can be solved with IGA. In fact, IGA has already been successfully applied across many areas of engineering application with great success.
- Isogeometric Analysis: Toward Integration of CAD and FEA.
J. Austin Cottrell, Thomas J. R Hughes, Yuri Bazilevs - Isogeometric finite element data structures based on Bézier extraction of NURBS.
Michael J. Borden, Michael A. Scott, John A. Evans, Thomas J. R. Hughes
IGA opens up new frontiers in simulation — The unique attributes of IGA and analysis-suitable CAD make it possible to attack next generation problems which are currently out of reach for traditional FEA tools.
- Tissue-scale, personalized modeling and simulation of prostate cancer growth.
Guillermo Lorenzo, Michael A. Scott, Kevin Tew, Thomas J. R. Hughes, Yongjie Jessica Zhang, Lei Liu, Guillermo Vilanova, and Hector Gomez - Isogeometric analysis of the Cahn–Hilliard phase-field model.
Héctor Gómez, Victor M. Calo, Yuri Bazilevs, Thomas J.R. Hughes - Isogeometric shell analysis with Kirchhoff–Love elements.
J. Kiendl, K.-U. Bletzinger, J. Linhard, R. Wüchner
Start a conversation
Have questions about Coreform or its products? We’d love to hear from you.